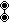Omnitruncation

Geometric operation

In geometry, an omnitruncation of a convex polytope is a simple polytope of the same dimension, having a vertex for each flag of the original polytope and a facet for each face of any dimension of the original polytope. Omnitruncation is the dual operation to barycentric subdivision.[1] Because the barycentric subdivision of any polytope can be realized as another polytope,[2] the same is true for the omnitruncation of any polytope.

When omnitruncation is applied to a regular polytope (or honeycomb) it can be described geometrically as a Wythoff construction that creates a maximum number of facets. It is represented in a Coxeter–Dynkin diagram with all nodes ringed.

It is a shortcut term which has a different meaning in progressively-higher-dimensional polytopes:

  • Uniform polytope truncation operators
    • For regular polygons: An ordinary truncation, t 0 , 1 { p } = t { p } = { 2 p } {\displaystyle t_{0,1}\{p\}=t\{p\}=\{2p\}} .
      • Coxeter-Dynkin diagram
    • For uniform polyhedra (3-polytopes): A cantitruncation, t 0 , 1 , 2 { p , q } = t r { p , q } {\displaystyle t_{0,1,2}\{p,q\}=tr\{p,q\}} . (Application of both cantellation and truncation operations)
      • Coxeter-Dynkin diagram:
    • For uniform polychora: A runcicantitruncation, t 0 , 1 , 2 , 3 { p , q , r } {\displaystyle t_{0,1,2,3}\{p,q,r\}} . (Application of runcination, cantellation, and truncation operations)
      • Coxeter-Dynkin diagram: , ,
    • For uniform polytera (5-polytopes): A steriruncicantitruncation, t0,1,2,3,4{p,q,r,s}. t 0 , 1 , 2 , 3 , 4 { p , q , r , s } {\displaystyle t_{0,1,2,3,4}\{p,q,r,s\}} . (Application of sterication, runcination, cantellation, and truncation operations)
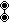
      • Coxeter-Dynkin diagram: , ,
    • For uniform n-polytopes: t 0 , 1 , . . . , n − 1 { p 1 , p 2 , . . . , p n } {\displaystyle t_{0,1,...,n-1}\{p_{1},p_{2},...,p_{n}\}} .

See also

References

  1. ^ Matteo, Nicholas (2015), Convex Polytopes and Tilings with Few Flag Orbits (Doctoral dissertation), Northeastern University, ProQuest 1680014879 See p. 22, where the omnitruncation is described as a "flag graph".
  2. ^ Ewald, G.; Shephard, G. C. (1974), "Stellar subdivisions of boundary complexes of convex polytopes", Mathematische Annalen, 210: 7–16, doi:10.1007/BF01344542, MR 0350623

Further reading

  • Coxeter, H.S.M. Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8 (pp. 145–154 Chapter 8: Truncation, p 210 Expansion)
  • Norman Johnson Uniform Polytopes, Manuscript (1991)
    • N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966

External links

Polyhedron operators
  • v
  • t
  • e
Seed Truncation Rectification Bitruncation Dual Expansion Omnitruncation Alternations
t0{p,q}
{p,q} 		t01{p,q}
t{p,q} 		t1{p,q}
r{p,q} 		t12{p,q}
2t{p,q} 		t2{p,q}
2r{p,q} 		t02{p,q}
rr{p,q} 		t012{p,q}
tr{p,q} 		ht0{p,q}
h{q,p} 		ht12{p,q}
s{q,p} 		ht012{p,q}
sr{p,q}